
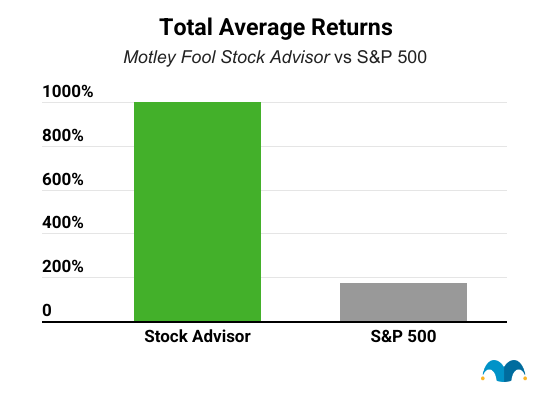
Five years from now, you'll probably wish you'd started investing with Stock Advisor
- New stock picks each month
- Detailed analysis of companies
- Model portfolios and advanced tools
- Live streaming during market hours
Featured Investing News


3 Reasons Royal Caribbean Stock Can Double Again in 2024

Dividend Watch: Your Move, Amazon

2 Stock-Split Stocks to Buy Hand Over Fist Right Now

Why Is Chipotle Stock the Largest Holding for Billionaire Bill Ackman?
Trending News
- Social Security's 2025 COLA Is on Track to Do Something It Hasn't Done For 15 Years
- 2 Stock-Split Stocks to Buy Hand Over Fist Right Now
- 1 Magnificent S&P 500 Dividend Stock Down 20% to Buy and Hold Forever
- 1 Unstoppable Stock That Could Join Microsoft, Apple, Nvidia, Alphabet, Amazon, and Meta in the $1 Trillion Club
- Billionaire Bill Ackman Has His Sights on Only 1 "Magnificent Seven" Stock, and It's Not Nvidia
- The Vanguard 500 Index Fund Is Great, But Another Vanguard ETF Has Outperformed It the Past Decade
- 1 Monster Artificial Intelligence (AI) Growth Stock Up 45,900% in 20 Years to Buy Now, According to Wall Street
- Some Retirees Will Get a $4,873 Check This Month From Social Security. Here's Why.
- Tesla Just Shared Some Spectacular News for Nvidia Stock Investors
- Where Will Rivian Stock Be in 5 Years?
- History Says the Nasdaq Will Roar Higher This Year. My Top Growth Stock to Buy Before It Does.
- 2 Roaring Growth Stocks to Hold for the Next 20 Years
- 2 Dividend Stocks to Double Up On Right Now
- Should You Buy Super Micro Computer Stock Before Tuesday?
- Claiming Social Security Spousal Benefits? Here's What the Average Senior in That Situation Collects Today.
Popular Topics
About The Motley Fool
Our Purpose: To make the world smarter, happier, and richer.
Founded in 1993 by brothers Tom and David Gardner, The Motley Fool helps millions of people attain financial freedom through our website, podcasts, books, newspaper column, radio show, and premium investing services. We believe that investing is empowering, enriching, and fun. We look forward to joining you on your journey to financial independence. Learn More
The Motley Fool Investing Philosophy
Learn How We Invest-
#1 Buy 25+ Companies Over Time
-
#2 Hold Stocks for 5+ Years
-
#3 Add New Savings Regularly
-
#4 Hold Through Market Volatility
-
#5 Let Your Winners Run
-
#6 Target Long-Term Returns
Investing Solutions Designed To Make Money Over The Long Term
Become a member today

Stock Advisor
Our base-level-membership giving you the foundation of a Foolish portfolio
Help members earn a positive return and build the foundations of a portfolio backed by Motley Fool principles.
Individuals who are seeking easy-to-use guidance on investing in more familiar-named companies.
$5,000 +
- Monthly stock recommendations (usually large and mid-cap stocks)
- Portfolio allocation guidance via The Motley Fool's Allocator tool
- On-going coverage of prior recommendations
- Access to Motley Fool Live video stream

Epic Bundle
A bundled membership that combines our four foundational stock-investing services into one
Help members outperform the benchmark by gaining exposure to a wider variety of stocks and investments.
Individuals who are regularly adding new money to their brokerage accounts, interested in business, and want to spend more time learning the art of investing.
$25,000 +
- Includes access to Stock Advisor + Rule Breakers + Everlasting Stocks + Real Estate Winners
- Weekly stock recommendations (ranging from small to large-cap stocks)
- Portfolio allocation guidance via The Motley Fool's Allocator tool
- On-going coverage of prior recommendations from all included services
- Access to Motley Fool Live video stream